Fair warning – this is a nitty-gritty article today. It’s got numbers and math, and you’ll know pretty quickly whether you 100% need to understand it, or if you’ll never care.
We’re going to describe a way to analyze mortgage options so you can determine which option is best for you.
But before we look at a specific real-life mortgage example, we need to understand net present value.
Aesop: The Original Discounter
To understand net present value (NPV), we must go back to Aesop. Finance, it turns out, loves Aesop.
- Investors know that the tortoise beats the hare. “Buy and hold” is the ultimate tortoise technique.
- Personal finance experts know about the ants and the grasshopper. The ants work hard and store food for bad times, whereas the grasshopper eats and splurges. And when bad times come, the grasshopper is screwed.
But I want to focus on the famous Aesopian saying: a bird in the hand is worth two in the bush. A bird right now, in other words, is the same as having two birds in the future.
Because the future isn’t guaranteed. Will the two birds be there when we need them?
And while waiting for the future, can we be productive with our current bird? Can we breed that bird into more than two future birds? Do we need to eat our current bird to simply survive for the future?
If you don’t believe what Aesop said, let me ask you this. Would you rather have:
- $1000 right now, in your hand, or
- $1000 in 10 years
Duh. It’s not a trick question. We’d all take the money right now.
If I ask you to wait 10 years for your money, you’ll charge me. That’s called a time premium. You’ll ask yourself questions like…
- Could I invest that $1000 today? If yes, what would it be worth in 10 years?
- Will inflation hurt the value of money over time? If yes, how much buying power will $1000 have in 10 years?
- Do I trust Jesse to actually have money to give me in the future? If not, how much “virtual interest” should I charge him for making me wait?
Those answers all point to a simple truth: $1000 right now is worth more than $1000 in the future.
$1000 in hand is worth more than $1000 in the bush.
Net Present Value (NPV)
Net present value applies Aesop’s wisdom to all sorts of financial scenarios. The world of finance is full of future dollars.
- Banks lend money today and expect repayment in the future.
- Investors buy assets today and expect cashflows in the future.
- Planners save money today and expect to spend it in the future.
In each scenario above, we need a system of comparing today’s money (e.g. a bank’s loan) to future money (the loan payments).
Net present value does that.
NPV calculations utilize discounting. (In fact, some people—like Warren Buffett—use the term “discounted cash flow,” or DCF, instead of NPV. These two acronyms describe the same process.)
Discounting is the mathematical process of reducing the value of future dollars. Thus, the “discount rate” is the key aspect of an NPV analysis. The discount rate measures how much less a future dollar is worth compared to a current dollar.
Let’s use a simple example…
How Buffett NPVs
Buffett, for example, is well-known for using the 30-Year U.S. Treasury Rate for his discount rate. The logic here is simple. If Warren Buffet is sitting on a billion dollars, he could:
- Option A: invests his dollars in a 30-Year U.S. Treasury, guaranteed to return 3.317% (as of this draft) per year of the next 30 years.
- Option B: invests his dollars in a particular deal he’s analyzing, with its unique costs and uncertain future cashflows.
Does the current deal (Option B) outperform the U.S. Treasury (Option A)? That’s the baseline question. If no, then why would he ever invest in this particular deal?
But then Buffett repeats the process for dozens, hundreds, or thousands of different “Option B”‘s. He’s looking for the sweetest, easiest investment he can find.
And it’s hard to compare these unique deals. They have different cashflows over different time periods. NPV solves that problem by discounting every future dollar into today’s dollars. That’s why Buffett discounts each “future dollar” from each Option B.
Quick NPV Example
If I guaranteed giving you $1000 today or $2000 in 10 years, which would you pick?
Well, if I discount $2000 by 3.317% for 10 years, I get an NPV of $1443.
$2000 / (1.03317 ^ 10) = $2000 / 1.386 = $1443
And since $1443 is greater than $1000, the math suggests going with the future $2000 option.
But some of you might ask…but, doesn’t the stock market return 10% per year (on average, never guaranteed). And even if inflation is 4% per year, that still leaves me with a discount rate of 6% per year, not 3.317% per year.
And at that discount rate, the future $2000 is only worth $1116 today. If I use a discount rate of 10%, the future $2000 is only worth $771 today.
We’ve hit upon the hardest problem in NPV analysis: what discount rate should we use, and why? Low discount rates make future dollars more appealing. High discount rates make current dollars more appealing.
Each NPV analysis has unique attributes that influence us to pick one rate over another. Some common choices include:
- The risk-free rate (usually U.S. government debt, hence Buffett’s choice of 30-year treasury rate)
- The hurdle rate, or MARR
- The borrowing rate (if you’re taking a loan)
- A combination of various rates, with the knowledge that some rates may change over time
Back to Mortgages
A friend-of-the-blog recently asked me to help him compare various mortgage options.
Mortgages combined a lump sum today (the down payment) with a series of future payments. Each unique mortgage has it’s own terms – interest rate, down payment, etc. How do we compare one mortgage to another?
We use NPV. We discount all the future payments into today’s dollars, and then determine which mortgage represents the least amount of debt.
To keep things simple, I’ll present two options here for a $300,000 home:
Option 1:
- 30-year term
- 20% down-payment
- 5.5% interest rate
- Various closing costs
Option 2:
- 30-year term
- 10% down-payment + $5000 to eliminate private mortgage insurance (PMI) payments
- 5.0% interest rate, secured after spending $10,000 on “mortgage points“
- Various closing costs
And to make matters more complicated, this person isn’t sure how long they’ll own the home for. Thankfully, NPV is capable of handling that uncertainty.
So we create a handy spreadsheet to calculate the loan payments over time, we discount those payments, and then we plot out the discounted value of the mortgage based on how long they’ll own the home.
- Here’s the spreadsheet.
- And here’s the graph of the NPV over time…
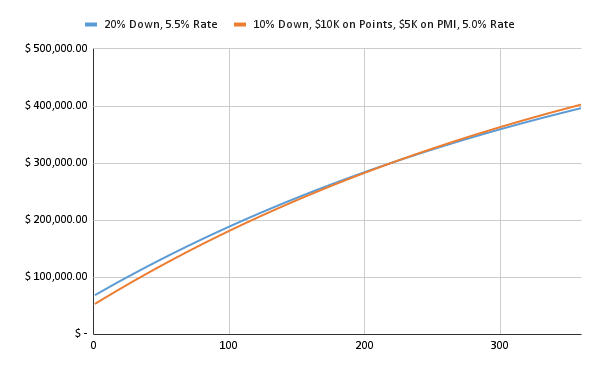
What takeaways do we have?
If this person is going to own the house for less that ~18.5 years, than the 10% down/$10K on points option makes the most sense. That option has the lower NPV over that period.
But if this is a “forever home” for 2+ decades, then the 20% down mortgage makes more sense.
If you’re interested in doing this analysis, but don’t feel comfortable, just reach out to me! I’d be happy to help.
Let’s Close on This Deal…
We can use real math and real financial tools to help us understand personal finance questions. Net present value is a terrific example of that.
A bird in the hand is worth two in the bush. And money today is worth more than money in the future.
Thank you for reading! If you enjoyed this article, join 8000+ subscribers who read my 2-minute weekly email, where I send you links to the smartest financial content I find online every week.
-Jesse
Want to learn more about The Best Interest’s back story? Read here.
Looking for a great personal finance book, podcast, or other recommendation? Check out my favorites.
Was this post worth sharing? Click the buttons below to share!